Pressure Field Equations

As seen in the last section we can conclude that the pressure at a single point is independent of direction when there are no shearing stresses present.  However, next we need to determine how the pressure of a fluid will vary from one point to the other when there is no shearing stresses present.  In essence creating a pressure field.

Let’s start by looking at a basic cubic fluid element.  On this element there are two different type of forces that are interacting with it.  They are surface forces and body forces. Surface forces are caused by the external pressures acting on the element.  While body forces consider the weight of the element caused by the specific weight of the fluid.

 

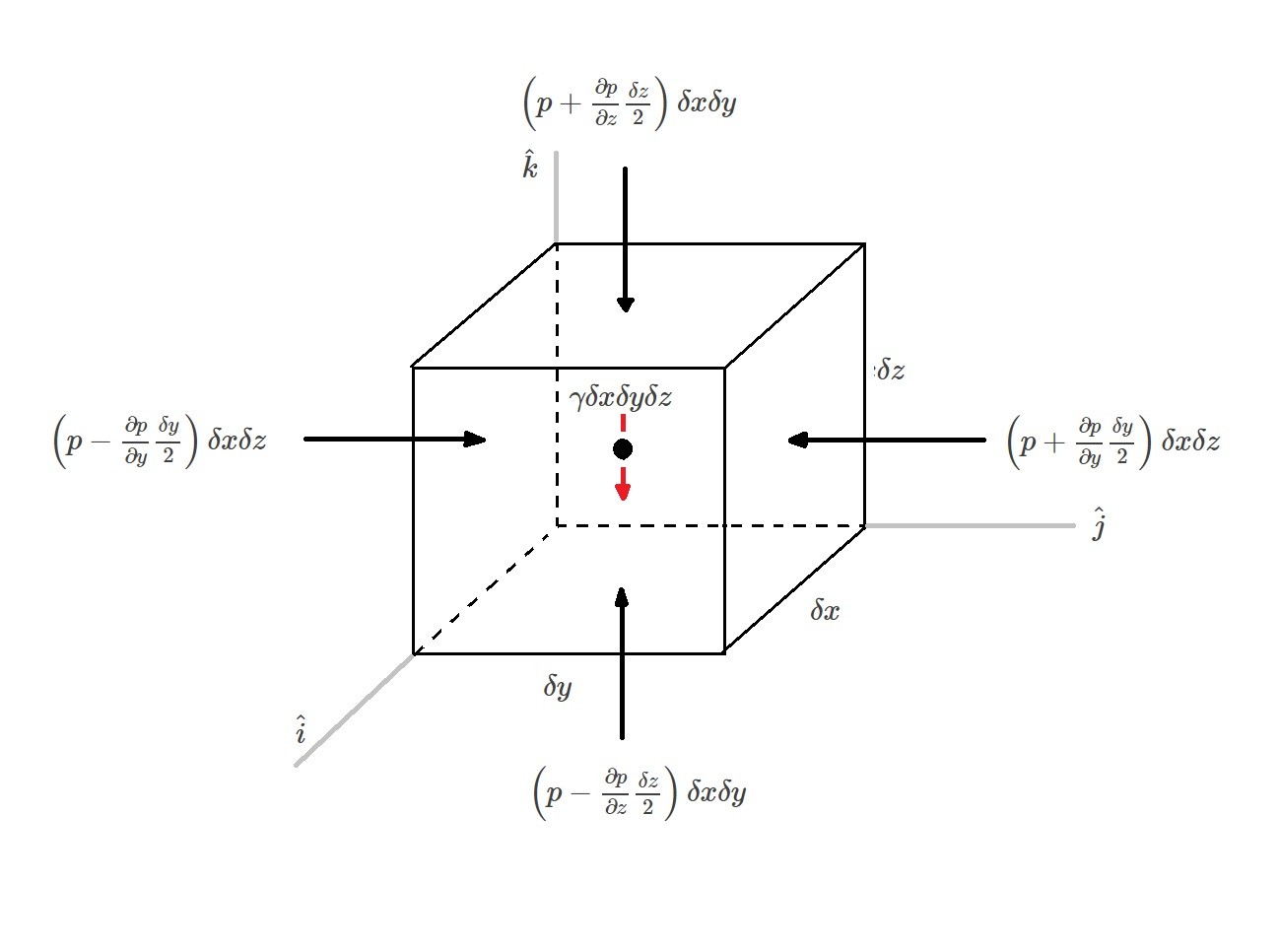
Surface Forces

First let take a look at the surface forces on the fluid element shown above. The different surface forces are caused by a pressure (p) on the face of the element.  The average pressure on each face is a product of the pressure and its derivative.  As δx, δy, and δz approach zero, higher order terms can be removed from the equation.  The equation below represents the resultant force in the y-direction.

(Eq 1)  $\delta F_y=(p-\frac{\partial p}{\partial y}\frac{\delta y}{2})\delta x\delta z-(p+\frac{\partial p}{\partial y}\frac{\delta y}{2})\delta x\delta z$

or

$\delta F_y=-\frac{\partial p}{\partial y}\delta x\delta y\delta z$

From the above the resultant forces in the x and z can be determined to be the following.

(Eq 2)  $\delta F_x=-\frac{\partial p}{\partial x}\delta x\delta y\delta z$

(Eq 3)  $\delta F_z=-\frac{\partial p}{\partial z}\delta x\delta y\delta z$

Finally, the above equations can be combined and expressed in vector form.

(Eq 4) $F_R=\delta F_x\hat{i}+\delta F_y\hat{j}+\delta F_z\hat{k}$

or

$\frac{\partial p}{\partial x}\hat{i}+\frac{\partial p}{\partial y}\hat{j}+\frac{\partial p}{\partial z}\hat{k}=\nabla p$

where

$\frac{\delta F_R}{\delta x\delta y\delta z}=-\nabla p$

Body Forces

Next, we are going to have to consider any body forces effecting the fluid element.  In this case the force would be the weight of the fluid element caused by the specific weight of the fluid.

(Eq 5) $-\delta W\hat{k}=\gamma \delta x\delta y\delta z\hat{k}$

$\gamma$ = specific weight

Finally, using Newton’s second law of motion $F=ma$  the surface forces and body forces of the fluid element can be combined.

(Eq 6) $-\nabla p–\gamma \hat{k}=\rho a$

$\rho$ = Fluid Density

$a$ = acceleration

Equation 6 represents the general equation of motion for a fluid element when there is no shear stresses present on the fluid element.

 

Previous  |  Next

⇑  |  ⇓